The universe is an expansive tapestry woven with intricate threads of matter and forces, among which black holes and neutron stars stand out as remarkable phenomena of cosmic evolution. Understanding their properties and the implications of their existence requires a deep dive into modern astrophysics and the principles of quantum mechanics. This article will explore the fundamental distinctions between these entities, their underlying physical laws, and the prevailing misconceptions that often cloud our understanding.
At the forefront of studying compact celestial bodies, neutron stars emerge as stellar remnants left after the explosion of supernovae. With an extraordinary density, a neutron star is packed so tightly that a sugar-cube-sized amount of its material would weigh about the same as a mountain on Earth. These stars possess a maximum mass estimated around 2.34 solar masses, regulated by the principles of quantum chromodynamics, which governs the strong nuclear force responsible for binding protons and neutrons.
The interplay of forces in neutron stars exemplifies the balance of gravity and quantum mechanics. This balance manifests in the form of the Pauli Exclusion Principle, which dictates that no two fermions, including the neutrons that constitute the star, can occupy the same quantum state. This principle acts as a counterbalance to the immense gravitational pull, preventing the star from collapsing into a black hole under normal circumstances.
In stark contrast to neutron stars, black holes are the ultimate enigma of astrophysics. Defined as acausal spacelike entities, black holes defy the conventional understanding of mass and gravity. Unlike neutron stars, which have a definitive maximum mass, black holes can absorb unlimited mass, resulting in configurations that can reach millions or even billions of solar masses. Their nature diverges significantly from timelike matter and raises questions about the fundamental principles underlying their formation and behavior.
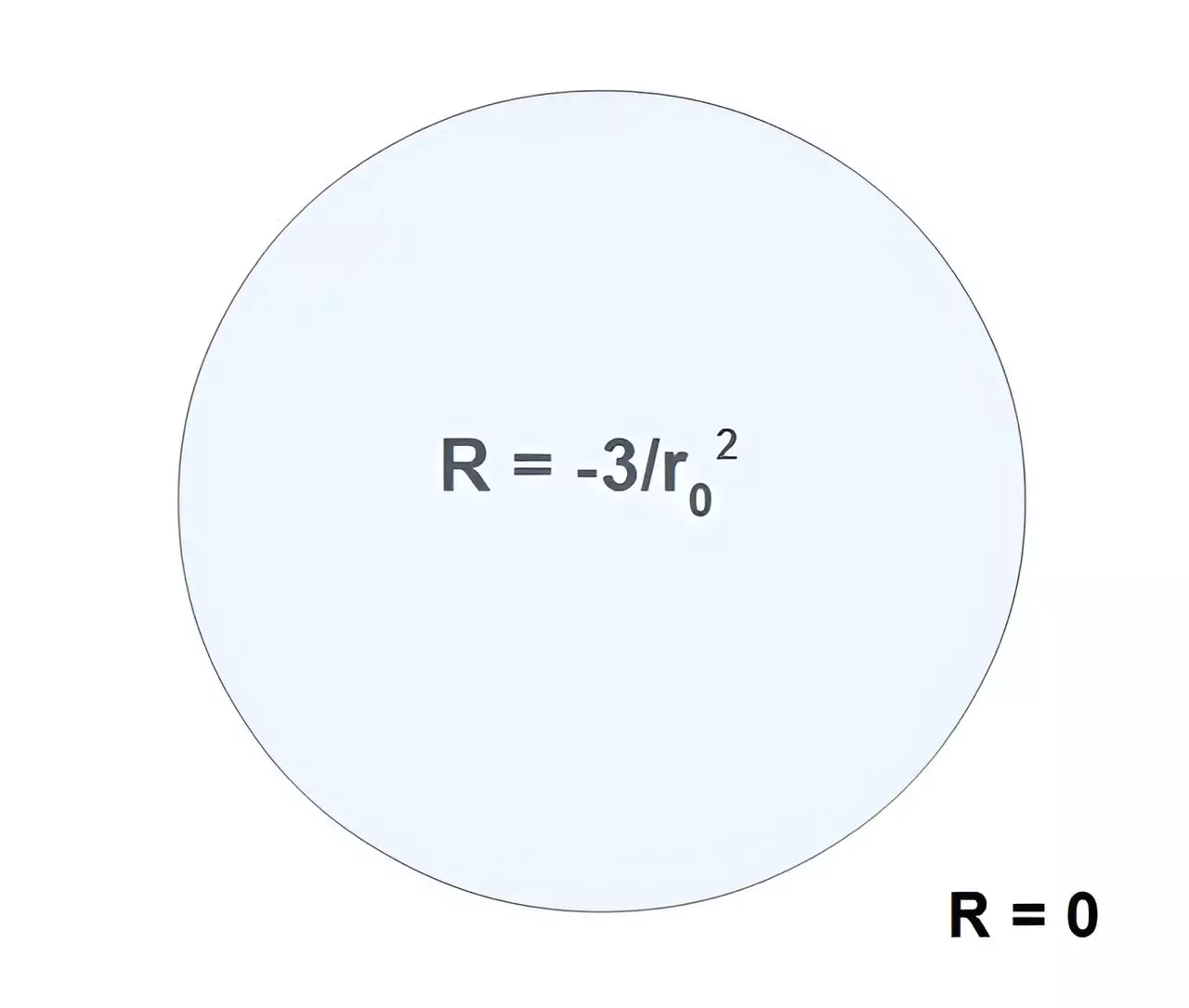
When delving into the physics of black holes, one discovers that they lack identifiable particle states and are characterized instead by gravitational invariants — quantities that retain their properties regardless of the observer’s perspective. The only metrics available to describe black holes encompass gravitational invariants like volume, area, radius, and scalar curvature. This is a crucial departure from conventional celestial bodies, which adhere to associative equations of motion and thermodynamic laws.
Recent studies propose the existence of a universal force constant that influences the dynamics of black holes, resulting in the relationship between outward and inward pressures described by ( P_S = -P_M ). This equilibrium leads to the conclusion that all black holes share a universal constant, ( F = 3c^4/4G ), reflective of the balance created by gravitational forces operating within them. This presents intriguing ramifications for our understanding of cosmic pressures, with black holes exhibiting the highest pressures in the universe despite substantial size differences.
With the smallest black hole estimated to have a pressure around ( 1.5183 times 10^{35} , N/m^2 ), the implications are astounding. For context, this value surpasses the core pressure of Jupiter significantly, challenging our grasp of cosmic phenomena and their extremes. The physics behind black holes thus exemplifies the interplay between significant theoretical propositions and observational data.
An exciting area of current research is the coalescence of black holes, guided by a specific area law fundamentally different from the conventional entropy laws proposed by Stephen Hawking. According to this new area law, when two black holes merge, the conditions dictated by their pressures must satisfy ( P_1 + P_2 > P_3 ). This principle not only beckons for a deeper understanding of gravitational wave emissions observed during such events but encourages revisiting established theories on black hole thermodynamics.
The intricate nature of these cosmic mergers and the associated gravitational waves urges astronomers and physicists to reconceptualize traditional understandings. By applying a reciprocal area relationship, ( frac{1}{A_1} + frac{1}{A_2} > frac{1}{A_3} ), new avenues for empirical research arise, promising a path toward elucidating the mysteries surrounding black holes.
Crucial to the advancement in understanding is recognizing the mistakes which arise when attempting to apply causal physics principles to acausal objects. Common claims regarding black holes often erroneously suggest that they harbor singularities, emerging from an incorrect application of Einstein’s equations. Furthermore, misconceptions surrounding black holes radiating and evaporating contradict foundational aspects of their negative scalar curvature.
This misalignment of theories emphasizes the need to re-evaluate longstanding assumptions regarding black hole thermodynamics. The nuances between causal and acausal entities demand careful consideration, challenging researchers to refine their approaches in this complex domain of physics.
The cosmos is replete with mysteries that continuously test the boundaries of human knowledge. Neutron stars and black holes serve not only as symbols of extreme physics but also as gateways leading to a broader understanding of the universe. As cosmologists strive to resolve the contradictions presented by acausal black holes, they unveil a deeper appreciation for the fundamental laws of nature. This journey into the complexity of cosmic phenomena proves both a challenge and an inspiration, driving the scientific community to further unravel the enigmas of the universe.